even permutation | Odd/Even Permutations

Card game based on Family Feud. Skip Navigation Accessibility Feedback. . Board Game; Family; Publisher; Person; Accessory; Podcast; Accessory Family; Award Set; Award Category; Periodical; . Family Feud Jumbo Card Game: Platinum Edition (2019) Card game based on Family Feud.

even permutation*******If the number of transpositions is even then it is an even permutation, otherwise it is an odd permutation. For example (132) is an even permutation as (132) = (13)(12) can be written as a product of 2 transpositions.

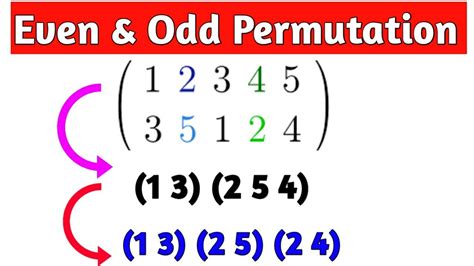
Learn the definition and examples of even and odd permutations, and the theorems related to their properties and operations. Find out how to identify and prove even and odd permutations using transpositions.

Learn the definition and examples of even and odd permutations, and the theorems related to their properties and operations. Find out how to identify and prove even and odd permutations using transpositions.Odd/Even Permutations Learn the definition and examples of even and odd permutations, and the theorems related to their properties and operations. Find out how to identify and prove even and odd permutations using transpositions.

the act or process of changing the linear order of an ordered set. [ 1] An example of the first meaning, is the six permutations (orderings) of the set {1, 2, 3}: written as tuples, they are (1, 2, 3), (1, 3, 2), (2, 1, 3), (2, 3, 1), (3, 1, 2), and (3, 2, 1).Even and Odd Permutations. Recall from the Inversions of Permutations page that if A = {1, 2,., n} is a finite n -element set of positive integers then an inversion of the n -permutation (x1,x2,.,xn) occurs when j < k and xj > xk.If G includes odd permutations, the even permutations form a proper subgroup that maps to 0 under parity, while the odd permutations map to 1. The even permutations form the kernel of the parity homomorphism, and are a normal subgroup in G.even permutation Odd/Even Permutations This video explains how to determine if a permutation in cycle notation is even or odd.two even or two odd permutations multiplied (i.e. composed) together give us an even permutation and an odd and an even permutation multiplied together give us an odd permutation. Example: (123)(45)(6789) is even since (123) = even, (45) = odd, and (6789) = odd, so even + odd + odd = even. We show how to determine if a permutation written explicitly as a product of cycles is odd or even.For example, the identity permutation \(\id = (1,2)(1,2)\) so it is even. It follows straight from the definition that an even permutation multiplied by another even permutation is even, even times odd is odd, odd times even is odd, and odd times odd is even. It’s not clear however that a permutation couldn’t be odd and even at the same time.

Every permutation of a finite set can be expressed as the product of transpositions. [43] Although many such expressions for a given permutation may exist, either they all contain an even number of transpositions or they all contain an odd number of transpositions. Thus all permutations can be classified as even or odd depending on this number.

A transposition is clearly an odd permutation. Composing a permutation with a single transposition alters the parity of the number of inversions. The product of two even permutations, and also of two odd ones, is an even permutation, while the product of an even and an odd permutation (in either order) is odd.

A permutation is called an even permutation if its expression as a product of disjoint cycles has an even number of even-length cycles. Alternatively, a permutation is called an even permutation if it can be written as a product of an even number of transpositions. These two definitions can be seen to be equivalent.

The sign of a permutation $\\sigma\\in \\mathfrak{S}_n$, written ${\\rm sgn}(\\sigma)$, is defined to be +1 if the permutation is even and -1 if it is odd, and is given by the formula $${\\rm sgn}(\\sigm.

Even permutation definition: . See examples of EVEN PERMUTATION used in a sentence.An even permutation is a permutation created by an even number of two-element swaps. If the list has length 9 or less, all even permutations will be returned. If the list has length longer than 9, a random sampling of even permutations will be returned. Examples. Example Notebook.

A permutation is an arrangement of objects in which the order of selection matters. Visit BYJU’S to learn permutation with and without repetition, formulas with many solved examples. Login. Study Materials. . The even positions .The identity permutation, {1,2,3,4,5}, has zero pairs out of order, hence it is even. The permutation {5,4,3,2,1} has ten pairs out of order, and is also even. If two adjacent elements are swapped, i.e. a transposition, the parity of the permutation is reversed. The swap only changes the pair in question; all other pairs are unaffected. In this video we explore how permutations can be written as products of 2-cycles, and how this gives rise to the notion of an even or an odd permutationPermutations of 4 elements Odd permutations have a green or orange background. The numbers in the right column are the inversion numbers （OEIS數列A034968）, which have the same parity as the permutation.. 在數學中，當X是一個至少有兩個元素的有限集合時，X的置換（即從X到X的雙射）可分為大小相同的兩類：奇置換與偶置換。

No permutation is both odd and even. $(123)$ is an even permutation. It is the cycle that sends $1\mapsto 2\mapsto 3\mapsto 1$.It is not the identity permutation. This cycle notation may be a bit confusing in this way if we also use two line notation, in that we also write the two line notation with parentheses and it means something completely different.even permutation On wikipedia: Proof 1 states that the identity permutation is an even permutation (which it is) then assumes that the identity permutation is not also an odd permutation (this is analogous to assuming that a closed set is not an open set). Even permutations are white: . the identity; eight 3-cyclesthree double-transpositions (in bold typeface)Odd permutations are colored: six transpositions (green) six 4-cycles (orange) The small table on the left shows the permuted elements, and inversion vectors (which are reflected factorial numbers) below them. Another column shows the inversion sets, .

$\begingroup$ The identity permutation is clearly even, since it’s the product of $0$ transpositions, and $0$ is even. If you’ve proved the theorem that every permutation is either even or odd, there’s no reason to repeat the proof for .

Overview. Emirates operates this 777-300ER aircraft model in three different configurations. This version operates in a two class layout. To verify the correct version of this aircraft at the time of booking or at online check-in, the following rows may be used as reference: Business Class are rows 1-6 and Economy begins row 8.

even permutation|Odd/Even Permutations